One word could summarize my interest in science: "Change." It may be a cliché to say nothing stays the same (although true), but the scientific study of change is a central preoccupation of physics and chemistry, with the word "dynamics" cropping up at every turn. And in the science of life and living organisms, "evolution"—the process whereby species change over time—is regarded by most as biology's greatest idea.
My own path to biology certainly required many changes. After nearly a decade as professor in the solid mechanics group at Brown University, I came to Caltech as an applied physicist with an eye to using the move as an opportunity to start something new. The idea was to pursue a long-standing interest in the living world, but to keep one foot squarely planted in my original field by approaching biological studies with the familiar languages of mathematics and physics. Speaking of his school days in his autobiography, Charles Darwin (1809–1882) noted, "in after years I have deeply regretted that I did not proceed far enough at least to understand something of the great leading principles of mathematics, for men thus endowed seem to have an extra sense."
But what is the meaning of Darwin's "extra sense"? None of us knows how to listen to a rainbow or see the sound of a musical note. What I think he meant is that the application of mathematics to science sometimes allows us to "see" things that would otherwise remain completely hidden. That passage from Darwin's autobiography reminds me of a similar story surrounding his famed British contemporaries Michael Faraday (1791–1867) and James Clerk Maxwell (1831–1879). Michael Faraday is widely considered one of the greatest experimentalists of all time. His path from bookbinder's apprentice in his late teens to a laboratory technician post at the Royal Institution in London, where he became the preeminent discoverer of his time, is remarkable—yet he had no formal training, least of all in mathematics. Decades after Faraday's important contributions to the subjects of electromagnetism and electrochemistry, Maxwell reformulated Faraday's ideas in the famous equations that bear his name. Maxwell's quantitative connection between light and electromagnetism is considered one of the great accomplishments of nineteenth-century physics. In a way, Maxwell used Darwin's "extra sense" to refashion Faraday's thinking in mathematical terms: "As I proceeded with the study of Faraday, I perceived that his method of conceiving the phenomena was also a mathematical one, though not exhibited in the conventional form of mathematical symbols." Maxwell effectively "translated" Faraday's ideas "into a mathematical form." Today, work in my group tries to follow the Faraday-Maxwell example in our own small way, but in a completely different setting: namely, biology.
One could argue that life science is engaged in a period of explosive growth that rivals or even outpaces that of the fields of physics and astronomy after the invention of the telescope. In our view, many biological discoveries are themselves conceived in a mathematical form, even if not expressed by traditional symbols. So our group aims to mathematicize cell biology. All our work is under the umbrella of physics meets biology—we are engineers and physicists who hope to bring fresh and useful insights to biology by thinking about biological problems mathematically.
We use several distinct case studies to illustrate that approach. Our work spans a range of problems—for example, we're looking at how bacteria respond to sugar or salt in their environment, how cells organize their massive genomes (in human cells, one meter of DNA is packed into the tiny nucleus, which has a size less than one-tenth the width of a human hair), and how viruses transfer their genetic material as they infect their hosts.
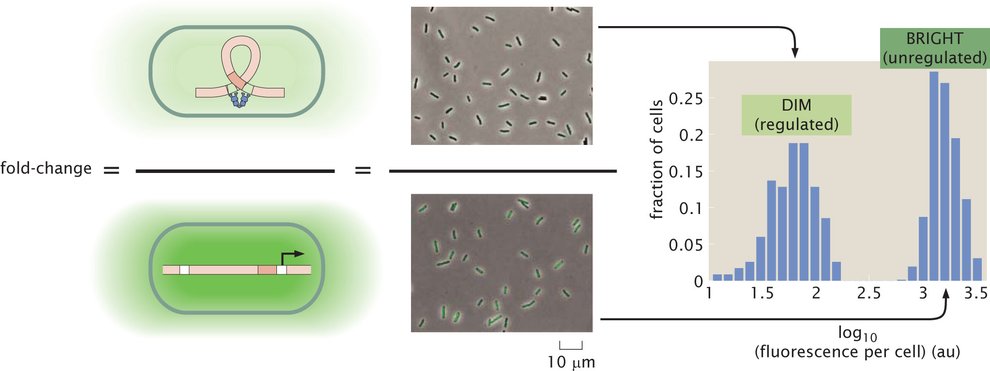
Credit: Rob Phillips. This figure shows how we measure how much a given gene is on by looking at how much green fluorescent light the cells give off. In fact, we measure the level of expression relative to a standard and construct their ratio to find a quantity we call the fold change. The brightness of the two populations of cells is seen in the two histograms on the right. In particular, the two peaked distributions show what fraction of the cells are "bright" and "dim," corresponding to those in which the gene of interest is unregulated and those in which that same gene is turned off.
We're exploring the use of mathematics as a criterion to determine whether the outcome of a given biological experiment is surprising. What our group does is address certain problems at the cellular level, and perform calculations to quantify our results. We are applying Darwin's "extra sense" in situations where we wouldn't actually know if we're surprised unless we quantify the results.
To illustrate, we're working on a problem that is already more than a hundred years old, which focuses on how microorganisms such as bacteria and yeast get the carbon they use to make new cells. In the late nineteenth century, studies on yeast fermentation raised many questions about how cells get their carbon. A more recent version of these questions focuses on how bacteria decide what to eat. Just as you and I might prefer doughnuts to broccoli, bacteria care about what carbon source you give them. If you give them several choices of sugar, they will choose only one. They will use the one source until they've exhausted it, and then they'll choose another. That is, they rank-order their carbon sources—and we're interested in the genes that control this choice. This classic problem resulted in the discovery of the modern theory of gene regulation—yet certain quantitative features are disturbing. That is, they don't make sense when viewed through a quantitative lens.
Some biologists may see this as unimportant—the basic work was already done in the 1970s—but I'm not so sure. Here's why: If you apply polymer physics to DNA, you immediately see that in some circumstances within living cells, DNA is forced into highly curved configurations. We're trying to work out how DNA gets bent and folded and crumpled up inside of cells, and how that activity affects gene regulation. This folding, in turn, is related to behavioral choices of the bacteria: The folding of the DNA has to do with what it chooses to eat (and decisions of many other kinds as well). When it doesn't want to eat lactose, DNA is folded in one way, and when it decides to eat lactose, the protein that folds it lets go and then it turns on the genes that make lactose digestion possible. We are trying to watch the cells' decisions in real-time, and to understand the consequences of flexibility of DNA.
This is where our approach parts with tradition: we first calculate, and then we measure. We measure both in vitro, meaning in the test tube, and at the same time, we measure in what some have christened the test tube of the twenty-first century: the cell itself. In other words, we behave first as theoretical physicists by applying calculations that describe what we know about how the gene networks in a bacterium work—what you would call the input/output relationship of the bacterium. We calculate how much of the enzyme needed to chop and eat a specific sugar is present for different cellular circumstances. Based upon differences related to gene circuit wiring, we calculate different input/output relations and produce a mathematical theory of the process. We then take that mathematical theory into the lab and redesign bacteria—that is, we actually "tune" the bacteria by altering the DNA. We go through the strands one by one, examining changes that result from the tuning process. We watch regulation by using a green fluorescent protein from jellyfish that has become a standard way of "reading out" cellular decisions. Our bacteria glow based on how much of the enzyme that digests sugar is present, and this tells us whether our calculations are "right" or not.
Often the most interesting cases are those in which something doesn't make sense, and for the problems we are attacking, to even know that things don't make sense you need a mathematical description of the problem. And this is how we know whether we're surprised: the differences are quantitative—a qualitative viewing of the problem is like trying to see the sound of a musical note.
Why do we study bacteria? A current trend in biology is toward the kind of cells that make up we humans, or eukaryotes—organisms whose cells contain complex structures enclosed within membranes. But in terms of numbers, eukaryotes represent a tiny minority of all living things. In a human body, there are ten times more microbes than human cells. If humans disappeared from Earth, the event would likely be a tiny hiccup in the overall scheme of things. If bacteria disappeared, the whole system would collapse. Indeed, Caltech has recently started an initiative on microbiology, led by Professor of Biology Dianne Newman. The collaborative effort includes researchers from a broad cross-section of the Caltech community who share a fascination with the microscopic living world.
But we also study bacteria because they are simple. The Nobel Prize–winning biologist Sydney Brenner is said to have quipped, "Either work on things that are six months ahead of everyone else, or 30 years behind." We are looking farther back than that, and our logic is this: In order to carry out the kind of systematic interplay between mathematical models and careful experiments described above, we must really "own" the systems. In the case of bacteria, we have in hand the necessary tools to "rewire" them as we might tune the resistances and capacitances in an electronic circuit. For example, when scientists and engineers create wiring diagrams of electronic circuits, they first must understand how individual components, such as a transistor or diode, works. Similarly, our work with bacteria, and E. coli in particular, is aided by the previous work of biologists who have figured out how to manipulate the wiring diagram. We use that work as our starting point and create precise mathematical forms of the various components that make up the genetic wiring diagram of the cell.
We are also interested in how bacteria react to changes in natural environments. An example of this is how osmoregulation works—the active regulation of the osmotic pressure of a cell's fluids to maintain the homeostasis of its water content. (Osmosis is the main way water is transported into and out of cells.) Just as our human bodies promote survival despite environmental insults such as cold temperatures, bacteria have similar mechanisms to preserve homeostasis in the face of changes in the osmotic state. Red blood cells respond by growing spikes under certain salt conditions, for example, and bacteria have reactions of their own to changes in their environment's salt content. If bacteria suddenly encounter a salt-free solution, water will rush in through their membrane, but they actually will still survive. This is part of a bacterium's normal life, and we're trying to understand how it works. Again, we start with our theoretical calculations and then perform single-cell measurements in a controlled environment. We do this by flooding our bacteria with pure water or various solutions of salt water and watching what happens to cells that are missing some of the proteins that rescue them during these osmotic shocks—essentially, they blow up. We measure that response according to the nature of each insult.
We are living through a historic time—scientists in the field of biology are making discoveries at a dizzying pace. In light of these advances, we and our colleagues at other institutions are becoming interested in building upon those discoveries by constructing simple but predictive models that not only provide a conceptual framework for explaining measurements that have already been done, but make polarizing predictions about experiments yet to be done. My friend and colleague, Tufts University School of Medicine Distinguished Professor, Emeritus, Moselio Schaechter, writes a blog called Small Things Considered. He recently asked what the apparent discovery of the particle known as the Higgs boson will do for biology. My own answer is that the study of the Higgs particle exemplifies the kind of interplay between theory and experiment that we should aspire to when describing biological systems, where surprising predictions are made and years of effort then follow to test them.
A new edition of my book Physical Biology of the Cell attempts to take stock of the great excitement surrounding efforts to mathematicize our understanding of biological systems. To capture this approach, we used images of calculations in the sand that depict our hopes for the kinds of calculations that one makes when first trying to mathematicize new problems. The images refer to a story about Archimedes of Syracuse (c. 287 BC–c. 212 BC), who is considered to be the greatest mathematician of antiquity and one of the greatest of all time. He is said to have died at the hands of a Roman soldier while performing calculations in the sand. His surviving works were an influential source of ideas for scientists. We are trying to illustrate calculations in biology that are simple enough to do with a stick in the sand. With that inspiration, the book explores the approach being taken by those working at the interface between biology and physics and shows how the basic tools and insights of physics and mathematics can illuminate the study of molecular and cell biology. We describe how quantitative models enable a better understanding of existing biological data and, most important, are the basis of unexpected predictions.
Yes, modern biology is complicated, but through the kinds of boundary-crossing, novel approaches seen in many divisions across the Caltech campus and elsewhere, I believe we will find surprising new ways of looking at important biological problems.
Rob Phillips is Fred and Nancy Morris Professor of Biophysics and Biology. Through independent study, he obtained his BS from the University of Minnesota in 1986. He received his PhD from Washington University in St. Louis in 1989. Following nearly a decade at Brown University and his 1997 appointment as Caltech's Clark Millikan Visiting Assistant Professor, he was appointed full professor at Caltech in 2000. He has held the Morris Chair since 2012. Professor Phillips focuses on physical biology of the cell: biophysical theory, single-molecule experiments, and single-cell experiments. Each summer, Professor Phillips hosts Bioengineering Bootcamp, a week-long, highly intensive course designed to introduce students to biological tools and techniques. The course places emphasis on the spatial and temporal scales associated with biological physics and also centers on molecular biology, gene expression, and subsequent protein translation in vivo. Students are also given the opportunity to work in groups on novel projects to learn about a particular biological system, experimental method, or biological material.

